Dissipation of the heat of natural underground water flows in the ground
The thermal dissipation, produced by the water arteries, grows blurred on very short distances - about the meter. It is thus not possible to detect those starting from thermal mesures taken on the surface. This thermal dissipation is, moreover, secondary compared to that which occurs within the aquifers (and which is not more detectable).
The geothermal reservoirs and the water arteries are not betrayed on the surface by thermic anomalies produced by the water arteries which do not end directly surfaces some, whatever the sensitivity of the instruments of detection used.
A quantitative illustration will clarify this fact. It is just about an example presented to give a direction of about size. The quantitative values differ from one situation to another, but the order of magnitude is sufficiently illustrative.
The radial variation of temperature between the axis of the water vessel and an unspecified point of the material (supposed to have a homogeneous conductivity thermal) which girds this water vessel is determined by the following equation:
![]()
where:
- λ is the thermal conductivity of material in W.m.C.-1-1
- φl is heat flow, by unit of length, from the water vessel in W.m or-1 J.m.s. -1-1
The values of temperature T and Tv, respectively associated with the rays R and Rv, are presented on the diagram below which illustrates the model of diffusion of heat around the water artery:
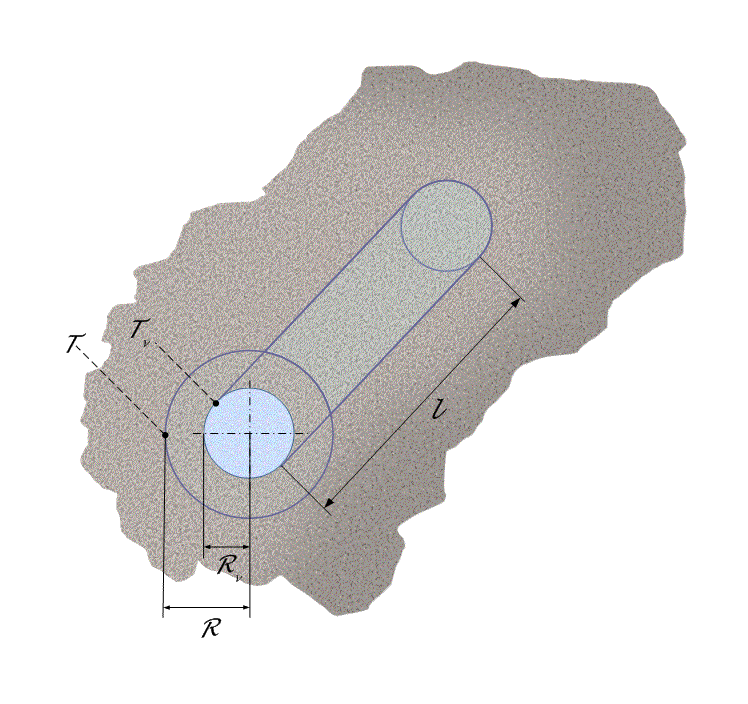
The effects edge at the ends are neglected. Let Us Suppose, for example, that the water artery - because it is this kind of vessel which interests us especially - shows the following characteristics:
- a 4,000 m length; it is about a rather long but at all exceptional water artery;
- a flow of 5 kg/s, it acts of a normal flow for a water artery. When a geothermal reservoir must evacuate a flow appréciablement higher, it produces a higher number of water arteries which form a spangled structure of branches known as cone (of cum natus, “born unit”);
- a temperature of the geological fluid of 350°C on the level of the exurgence point (which would be located typically at a depth between 1,000 and 2,000 meters);
- a temperature about 90° C of the lithospheric context of the exurgence point to “certain outdistances” water artery (about several meters);
- a differential of temperature over the entire length of flow of 250 °C; the water fluid thus has a temperature about 100°C to the place where it flows in an aquifer (i.e. at its point of emergence, which would be located typically at a depth of a few tens of meters);
- a temperature about 25° C of the lithospheric context of the point of emergence, to “certain distance” aquifer” in which flows the water artery;
- a thermal conductivity, λ, ground which varies according to the crossed rocks, but range typically between 0.8 and 2.2 W.m.°C-1. -1 Let Us Take 2 W.m.°C-1-1 to select a value of rather high conductivity of the crossed component lithic.
Heating capacity of the water being of 4,186 J·kg-1·°C-1, heat flow per unit of length, φl, generated by this hydrothermal flow is of:
φl =4.186 J.kg.°C-1 X-1 5 kg.s X 250-1 °C/4,000 m = 1,308 W.m. -1
The heat loss comes, at the same time, of the thermal energy of the geological fluid and frictions of the geological fluid on its walls of contact. While postulating that geothermal energy can be converted into electrical energy with a thermal efficiency of 14 %, the water artery produces a heat loss which would make it possible - if it were transformed into electricity - to feed more than 3 lamps of 60W per gone through meter.
Near the exurgence point (i.e. with the starter of the artery of the artery), the variation in temperature between the fluid geological (Tv, with 350°C) and its lithospheric context (T, with 90°C) is worth, for the digital illustration chosen, 260°C. This differential of temperature makes it possible to calculate the distance to which the temperature joined that of its environment by application of the equation of radial thermal diffusion process. This distance is about:
R ex ≈ 12.Rv
That means that the thermic anomaly is reabsorbed at a distance of about 12 times the ray of the water artery (that is to say less than 2 meters for a ray of about 15 cm), to as much say anything the whole compared to the depths from 1 to 2 km where this phenomenon occurs.
Near the point of emergence (i.e. at the end of the water artery), the variation in temperature between the fluid geological (Tv, with 100°C) and its lithospheric context (T, with 25°C) is worth, for the digital illustration chosen, 75°C. Such a variation in temperature is reabsorbed at a distance about twice that of the ray of the water artery.
R ém ≈ 2.Rv
Therefore, the water arteries are never thermically visible surfaces some if they do not arrive there directly. Even the most sensitive instruments infra-red cannot detect their presence.
